Difference between revisions of "Thermal Conductivity of Metals"
(Continuação do texto) |
m (Text replacement - "\]" to "</math>") |
||
| (14 intermediate revisions by 4 users not shown) | |||
| Line 1: | Line 1: | ||
| − | |||
| − | |||
=Description of the Experiment= | =Description of the Experiment= | ||
This experiment revolves around the conduction of heat. The user can study and compare how fast heat propagates through three bars of different materials. | This experiment revolves around the conduction of heat. The user can study and compare how fast heat propagates through three bars of different materials. | ||
| + | |||
| + | |||
| + | <div class="toccolours mw-collapsible mw-collapsed" style="width:420px"> | ||
| + | '''Links''' | ||
| + | <div class="mw-collapsible-content"> | ||
| + | |||
| + | *Video: rtsp://elabmc.ist.utl.pt:554/radiare.sdp | ||
| + | *Laboratory: Advanced in e-lab.ist.eu[http://e-lab.ist.eu] | ||
| + | *Control room: [Unavaliable] | ||
| + | *Level: **** | ||
| + | |||
| + | </div> | ||
| + | </div> | ||
| + | |||
<swf height="200" width="500">http://www.elab.tecnico.ulisboa.pt/anexos/descricoes-flash/ConducaoCalorFinal.swf</swf> | <swf height="200" width="500">http://www.elab.tecnico.ulisboa.pt/anexos/descricoes-flash/ConducaoCalorFinal.swf</swf> | ||
| Line 13: | Line 25: | ||
# Comparison of the output of each bar's first sensor. | # Comparison of the output of each bar's first sensor. | ||
| − | Each bar has a 5mm diameter and | + | Each bar is 370mm long and has a 5mm diameter and each one is made of a different metal: iron, copper and brass. |
| − | |||
| − | |||
| − | + | [[File:Conducao-montagem.jpg|thumb|Montagem: Mineral wool sandwich covering the metal cylinders.]] | |
| − | + | The first 70mm of each bar are wrapped with an heating resistor which occupies 2,5mm on it's center. In the opposite side is placed a refrigerated heat sink to keep this end at room temperature and is the position axis reference point. 10mm away from the heat sink is the first batch of thermometers, the second being at 160mm and the third at 210mm. The bars are mounted in the middle of two 50mm thick layers of thermal insulation material (mineral wool) that prevent heat convection and minimize thermal losses. | |
| − | + | In a pulsed or periodic regime, an electric current is applied to the heating resistor that heats that end through Joule's effect. The heat generated will run through the bar and will be dissipated at the opposite end in the sink. | |
| + | By choosing the pulse width (it should be around one minute), heat propagation can be observed by measuring the three points described above. | ||
=Protocol= | =Protocol= | ||
Run the experiment in pulse mode and observe how the pulse travels along the bars. | Run the experiment in pulse mode and observe how the pulse travels along the bars. | ||
| − | '''Note:''' The heat sink keeps the ends of the bars at room temperature, which means that the readings of one of the thermometers will not vary, having been chosen as the reference point | + | '''Note:''' The heat sink keeps the ends of the bars at room temperature, which means that the readings of one of the thermometers will not vary, having been chosen as the distance reference point. |
=Advanced Protocol= | =Advanced Protocol= | ||
| − | In | + | In the periodic mode, the temperature reading has two components: |
# one comes from the oscillation of the heating itself, its period being the same as the heat source (on and off); | # one comes from the oscillation of the heating itself, its period being the same as the heat source (on and off); | ||
| Line 40: | Line 51: | ||
For best results, please follow the procedure: | For best results, please follow the procedure: | ||
| − | # Execute two experiments in | + | # Execute two experiments in periodic mode, selecting a time interval of a few seconds between samples and a heating time of approximately one minute. For the first run, choose the maximum acquisition time possible, and for the second choose a reasonable value based on the data from the first one. |
| − | # Copy | + | # Copy the data to a spreadsheet or preferably to a science application, like Matlab or Origin; |
# Fit a math function to the thermal behavior of the bar; | # Fit a math function to the thermal behavior of the bar; | ||
| − | # | + | # Determine the period of oscillation for each experiment conducted and the phase shift between two consecutive thermometers, for each metal available; |
| − | # | + | # Numerically adjust the function to the experimental data to get the highest precision possible. |
| − | + | ||
| + | The thermal conductivity of each metal can be determined from the experimental data by using: | ||
| + | |||
| + | <math> | ||
| + | k = \frac{C_p \rho n \omega (x_1 - x_2)^2}{2 (\phi_1 - \phi_2) ln(A_1/A_2)} | ||
| + | </math> | ||
| + | |||
| + | where \( C_p \) is the metal's heat capacity, \( \rho \) the density, \( \omega \) the angular frequency, \( (x_2 - x_1) \) the distance between thermometers, \( (\phi_1 - \phi_2) \) phase between signals (measured in radians) and \( A_1/A_2 \) the ratio between amplitudes. | ||
| + | |||
| + | We can also calculate the ''k'' experimental uncertainty by using propagation formulas. | ||
| + | Note: the diameter of the bars was measured up to a ''0.01 mm'' and the sensor position up to ''0.5 mm''. | ||
| + | |||
| + | Afterwards, compare it to the textbook value. | ||
| + | |||
| + | A simple analysis can be done to check the experimental data, assuming the initial stimulus is sinusoidal. This way we can take ''n=1'' and the phase shift is determined by the distance between the maximum of the two experimental curves. | ||
| + | |||
| + | {| border="1" style="text-align: center;" | ||
| + | |+ Experimental results for different metals | ||
| + | |- | ||
| + | ! scope="col" | Metal | ||
| + | ! scope="col" | Specific heat<br /> \( C_p [J K^{-1} kg^{-1}] \) | ||
| + | ! scope="col" | Density<br /> \( \rho [kg m^{-3}] \) | ||
| + | ! scope="col" | Thermal conductivity<br /> \( k [W m^{-1} K^{-1}] \) | ||
| + | |- | ||
| + | |Brass | ||
| + | |384 | ||
| + | |8500 | ||
| + | |113 | ||
| + | |- | ||
| + | |Copper | ||
| + | |394 | ||
| + | |8930 | ||
| + | |390 | ||
| + | |- | ||
| + | |Iron | ||
| + | |456 | ||
| + | |7860 | ||
| + | |81 | ||
| + | |} | ||
| + | |||
| + | =Links= | ||
| + | *[[Determinação da Condutividade Térmica em Metais | Portuguese Version (Versão em português)]] | ||
Latest revision as of 21:50, 24 May 2015
Contents
Description of the Experiment
This experiment revolves around the conduction of heat. The user can study and compare how fast heat propagates through three bars of different materials.
Links
- Video: rtsp://elabmc.ist.utl.pt:554/radiare.sdp
- Laboratory: Advanced in e-lab.ist.eu[1]
- Control room: [Unavaliable]
- Level: ****
<swf height="200" width="500">http://www.elab.tecnico.ulisboa.pt/anexos/descricoes-flash/ConducaoCalorFinal.swf</swf>
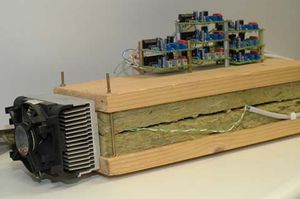
Experimental Apparatus
Two methods are used to study heat conduction through metals:
- Single thermal pulse, applied to both ends, and subsequent evolution through the three monitored points;
- Comparison of the output of each bar's first sensor.
Each bar is 370mm long and has a 5mm diameter and each one is made of a different metal: iron, copper and brass.
The first 70mm of each bar are wrapped with an heating resistor which occupies 2,5mm on it's center. In the opposite side is placed a refrigerated heat sink to keep this end at room temperature and is the position axis reference point. 10mm away from the heat sink is the first batch of thermometers, the second being at 160mm and the third at 210mm. The bars are mounted in the middle of two 50mm thick layers of thermal insulation material (mineral wool) that prevent heat convection and minimize thermal losses.
In a pulsed or periodic regime, an electric current is applied to the heating resistor that heats that end through Joule's effect. The heat generated will run through the bar and will be dissipated at the opposite end in the sink.
By choosing the pulse width (it should be around one minute), heat propagation can be observed by measuring the three points described above.
Protocol
Run the experiment in pulse mode and observe how the pulse travels along the bars.
Note: The heat sink keeps the ends of the bars at room temperature, which means that the readings of one of the thermometers will not vary, having been chosen as the distance reference point.
Advanced Protocol
In the periodic mode, the temperature reading has two components:
- one comes from the oscillation of the heating itself, its period being the same as the heat source (on and off);
- the other is the average heating of the bar as a whole, which is almost exponential.
Through a graphical fitting of a function to the average temperature, we can extract the oscillating values by subtracting the average. By analyzing the oscillating data, we can determine the heat propagation constant by Fourier analysis or a simple sinusoidal fitting.
For best results, please follow the procedure:
- Execute two experiments in periodic mode, selecting a time interval of a few seconds between samples and a heating time of approximately one minute. For the first run, choose the maximum acquisition time possible, and for the second choose a reasonable value based on the data from the first one.
- Copy the data to a spreadsheet or preferably to a science application, like Matlab or Origin;
- Fit a math function to the thermal behavior of the bar;
- Determine the period of oscillation for each experiment conducted and the phase shift between two consecutive thermometers, for each metal available;
- Numerically adjust the function to the experimental data to get the highest precision possible.
The thermal conductivity of each metal can be determined from the experimental data by using:
[math] k = \frac{C_p \rho n \omega (x_1 - x_2)^2}{2 (\phi_1 - \phi_2) ln(A_1/A_2)} [/math]
where \( C_p \) is the metal's heat capacity, \( \rho \) the density, \( \omega \) the angular frequency, \( (x_2 - x_1) \) the distance between thermometers, \( (\phi_1 - \phi_2) \) phase between signals (measured in radians) and \( A_1/A_2 \) the ratio between amplitudes.
We can also calculate the k experimental uncertainty by using propagation formulas. Note: the diameter of the bars was measured up to a 0.01 mm and the sensor position up to 0.5 mm.
Afterwards, compare it to the textbook value.
A simple analysis can be done to check the experimental data, assuming the initial stimulus is sinusoidal. This way we can take n=1 and the phase shift is determined by the distance between the maximum of the two experimental curves.
| Metal | Specific heat \( C_p [J K^{-1} kg^{-1}] \) |
Density \( \rho [kg m^{-3}] \) |
Thermal conductivity \( k [W m^{-1} K^{-1}] \) |
|---|---|---|---|
| Brass | 384 | 8500 | 113 |
| Copper | 394 | 8930 | 390 |
| Iron | 456 | 7860 | 81 |